GDS to PA:- 50 MCQ on Average
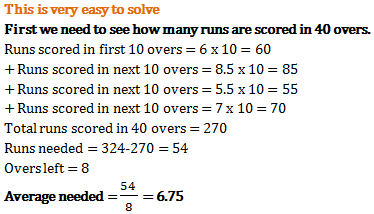
1. In the India-Australia one day match, due to rain, India needed 324 runs in 48 overs to win. In initial 10 overs, the average scoring rate was 6, but in next 10 overs it increased to 8.5. It then declined to 5.5 in next 10 overs and again rose to 7 in next 10 overs. To win the match now what average is needed?
A. 8.25
B. 6.75
C. 7.75
D. 7.00
... Answer is B)
A. 8.25
B. 6.75
C. 7.75
D. 7.00
... Answer is B)

2. Average age of 7 family members is 75 years. But average age of 6 of them is 74 years 6 months. What is the age of the 7th family member?
A. 75.5
B. 78
C. 68
D. 80
... Answer is B)
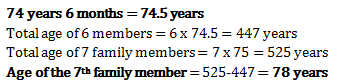
A. 75.5
B. 78
C. 68
D. 80
... Answer is B)
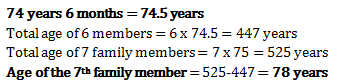
3.Average age of 5 people is 42 years. Another group has 8 people who have average age of 81 years. When both groups are mixed what is average age of all people?
A. 64 years
B. 66 years
C. 61.5 years
D. 70 years
... Answer is B)
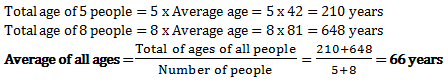
A. 64 years
B. 66 years
C. 61.5 years
D. 70 years
... Answer is B)
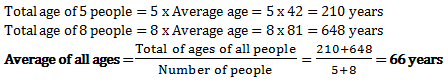
4) Average age of 5 people in a family is 55 years. However it is seen that 3 of the 5 people also have an average age of 55 years. What will be the average age of remaining two people of the family?
a. 82.5 years
b. 27.5 years
c. 55 years
d. 110 years
... Answer is C)
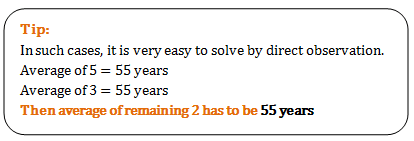
a. 82.5 years
b. 27.5 years
c. 55 years
d. 110 years
... Answer is C)
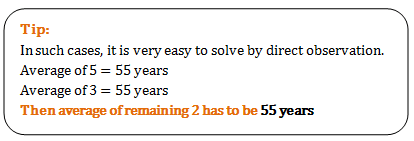
5.The average of fifty numbers is 28. If two numbers, namely 25 and 35 are discarded, the average of the remaining numbers is nearly,
a. 29.27
b. 27.92
c. 27.29
c. 27.29
... Answer is B)
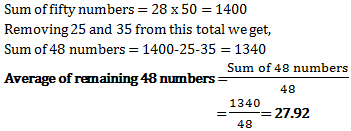
a. 29.27
b. 27.92
c. 27.29
c. 27.29
... Answer is B)
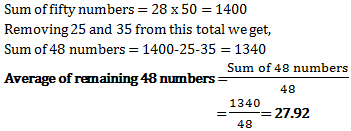
6.The average of three numbers is 77. The first number is twice the second and the second number is twice the third. Find the first number. The average of three numbers is 77. The first number is twice the second and the second number is twice the third. Find the first number.
,
a. 33
b. 66
c. 77
D. 132
... Answer is D)

a. 33
b. 66
c. 77
D. 132
... Answer is D)

7.Average age of A and B is 30 years, that of B and C is 32 years and the average age of C and A is 34 years. The age of C is
,
a. 33 years
b. 34 years
c. 35 years
D. 36 years
... Answer is D)
<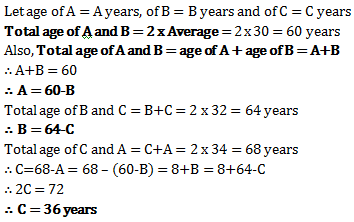
a. 33 years
b. 34 years
c. 35 years
D. 36 years
... Answer is D)
<
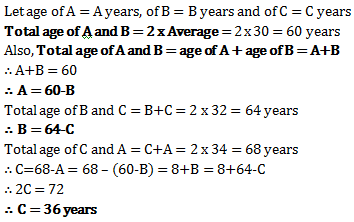
8. 3 boxes have some average weight. When one box which weighs 89 kg is replaced by another box, the average weight increases by 5 kg. How much the new box weight?.
,
A. 109kg
B. 94kg
C. 104kg
D. 94kg
... Answer is C)

A. 109kg
B. 94kg
C. 104kg
D. 94kg
... Answer is C)

9. Knowing that Vijay’s expenditure for first 3 days is Rs. 100, Rs. 125 and Rs. 85, what is his 4th day expenditure as his 4 days average expenditure Rs. 90?
,
A. Rs. 220
B. Rs. 60
C. Rs. 50
D. Rs. 90
... Answer is C)

A. Rs. 220
B. Rs. 60
C. Rs. 50
D. Rs. 90
... Answer is C)

11.Without considering the salary of the boss, the average salary reduces by Rs. 1000. What will be salary of boss if average salary of 11 employees and the boss is Rs. 18000?
,
A. Rs. 30000
B. Rs. 27000
C. Rs. 36000
D. Rs. 29000
... Answer is D)
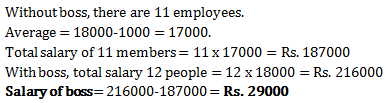
A. Rs. 30000
B. Rs. 27000
C. Rs. 36000
D. Rs. 29000
... Answer is D)
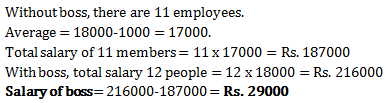
12. A batsman played 11 innings and has a certain average. This average increases by 2 runs when his 3 innings of 32 runs, 33 runs and 34 runs are replaced by 3 other innings. Find the average of these 3 new innings,
A. 40 runs
B. 35 (3/8) runs
C. 42 (3/8) runs
D. 40 (1/3) runs
... Answer is D)
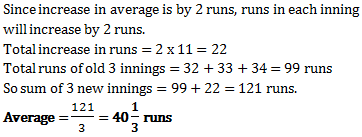
A. 40 runs
B. 35 (3/8) runs
C. 42 (3/8) runs
D. 40 (1/3) runs
... Answer is D)
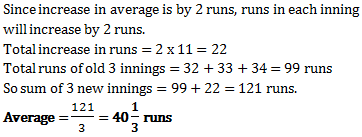
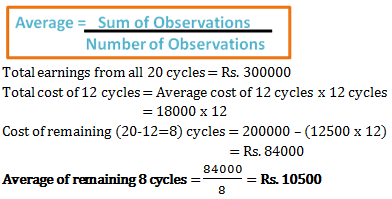
13.Of the 20 cycles sold by Ajay, average cost of 12 cycles is Rs. 18000. In total he earned Rs. 300000. What was the average cost of remaining cycles?
,
A. Rs. 10500
B. Rs. 7500
C. Rs. 9125
D. Rs. 9750
... Answer is A )
A. Rs. 10500
B. Rs. 7500
C. Rs. 9125
D. Rs. 9750
... Answer is A )

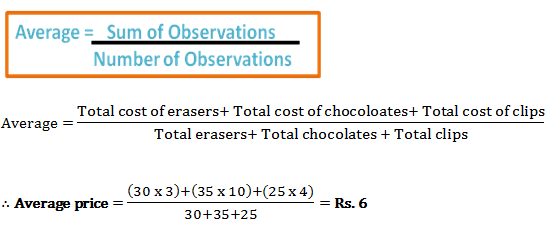
14.What will be average price of all the goods bought, if Ajay buys 30 erasers for Rs. 3 each, 35 chocolates for Rs. 10 each and 25 clips at the rate of Rs. 4 each?,
A. Rs. 9
B. Rs. 6
C. Rs. 5.5
D. Rs. 8.25
... Answer is B )
A. Rs. 9
B. Rs. 6
C. Rs. 5.5
D. Rs. 8.25
... Answer is B )

15.What is average of 5 runs, 8 runs, 11 runs, …., 26 runs, 29 runs and 32 runs?
,
A. 15.5
B. 27
C. 31
D. 18.5
... Answer is D )

A. 15.5
B. 27
C. 31
D. 18.5
... Answer is D )

16.In a race, average speed of total 75 bikes is 35km/hr. The average speed of red bikes is 55 km/hr. If average speed of green bikes is 30km/hr, then how many green bikes are there?
,
A. 70
B. 60
C. 45
D. 50
... Answer is B )

A. 70
B. 60
C. 45
D. 50
... Answer is B )

17. Find average of natural numbers from 1 to 65?
,
A. 33
B. 32.5
C. 130
D. 65
... Answer is A )

A. 33
B. 32.5
C. 130
D. 65
... Answer is A )

18. The average marks obtained by 100 candidates in an examination is 50. Find how many candidates have passed in the examination, if the average marks of candidates who passed is 70 and that of failed candidates is 20.
,
A. 90
B. 75
C. 60
D. 58
... Answer is C )
<"Let the number of students who passed the examination be X Number of students failed = (100 - X) Total marks of students who have passed = 70X Total marks of 100 students = 100 * 50 = 5000 Total marks of students who have failed = 20(100 – X) 20(100 – X) + 70X = 5000 2000 – 20 X + 70X = 5000 50 X = 3000 X = 60">
A. 90
B. 75
C. 60
D. 58
... Answer is C )
<"Let the number of students who passed the examination be X Number of students failed = (100 - X) Total marks of students who have passed = 70X Total marks of 100 students = 100 * 50 = 5000 Total marks of students who have failed = 20(100 – X) 20(100 – X) + 70X = 5000 2000 – 20 X + 70X = 5000 50 X = 3000 X = 60">
19. A grocer has a sale of Rs. 5420, Rs. 5660, Rs. 6200, Rs. 6350 and Rs. 6500 for 5 consecutive months. Find the sale he should have in the sixth month, so that he gets an average sale of Rs. 6000?
,
A. Rs. 5870
B. Rs. 5991
C. Rs. 6020
D. Rs. 6850
... Answer is A )
Explanation: Total sale for 5 months = Rs. (5420 + 5660 + 6200 + 6350 + 6500) = Rs. 30,130 Therefore, required sale = Rs. [(6000 * 6) – 30,130] = Rs. (36000 – 30,130) = Rs. 5870
A. Rs. 5870
B. Rs. 5991
C. Rs. 6020
D. Rs. 6850
... Answer is A )
Explanation: Total sale for 5 months = Rs. (5420 + 5660 + 6200 + 6350 + 6500) = Rs. 30,130 Therefore, required sale = Rs. [(6000 * 6) – 30,130] = Rs. (36000 – 30,130) = Rs. 5870
20.Ashok secured average of 75 marks in 6 subjects. If the average of marks in 5 subjects is 74, how many marks did he secure in the 6th subject?
,
A. 66
B. 74
C. 78
D. 80
... Answer is D )
Explanation: Number of subjects = 6 Average of marks in 6 subjects = 75 Therefore total marks in 6 subjects = 75 * 6 = 450 Now, No. of subjects = 5 Total marks in 5 subjects = 74 * 5 = 370 Therefore marks in 6th subject = 450 – 370 = 80
A. 66
B. 74
C. 78
D. 80
... Answer is D )
Explanation: Number of subjects = 6 Average of marks in 6 subjects = 75 Therefore total marks in 6 subjects = 75 * 6 = 450 Now, No. of subjects = 5 Total marks in 5 subjects = 74 * 5 = 370 Therefore marks in 6th subject = 450 – 370 = 80
21. The average temperature of a city in the first four days of a month was 36°. The average for the 2nd, 3rd, 4th and 5th day was 38°. If the temperature of the 1st and 5th day were in the ratio 4 : 5, then what is the temperature on the fifth day ?
,
A. 32°
B. 35°
C. 38°
D. 40°
... Answer is D
Explanation: The temperature for first 4 days = 4 * 36 = 144 The temperature for last 4 days = 4 * 38 = 152 The difference between the temperatures is (152 – 144) = 8 The ratio of temperature between first and fifth day is 4 : 5 The temperature on fifth day is 5*8 = 40
A. 32°
B. 35°
C. 38°
D. 40°
... Answer is D
Explanation: The temperature for first 4 days = 4 * 36 = 144 The temperature for last 4 days = 4 * 38 = 152 The difference between the temperatures is (152 – 144) = 8 The ratio of temperature between first and fifth day is 4 : 5 The temperature on fifth day is 5*8 = 40
22. Amol secured average of 75 marks in 6 subjects. If the average of marks in 5 subjects is 74, how many marks did he secure in the 6th subject?
,
A. 66
B. 74
C. 78
D. 80
... Answer is D
Explanation: Number of subjects = 6 Average of marks in 6 subjects = 75 Therefore total marks in 6 subjects = 75 * 6 = 450 Now, No. of subjects = 5 Total marks in 5 subjects = 74 * 5 = 370 Therefore marks in 6th subject = 450 – 370 = 80
A. 66
B. 74
C. 78
D. 80
... Answer is D
Explanation: Number of subjects = 6 Average of marks in 6 subjects = 75 Therefore total marks in 6 subjects = 75 * 6 = 450 Now, No. of subjects = 5 Total marks in 5 subjects = 74 * 5 = 370 Therefore marks in 6th subject = 450 – 370 = 80
23. The average runs of a cricket player of 10 innings was 20. How many runs must he make in his next innings so as to increase his average of runs by 4?
,
A. 12
B. 42
C. 64
D. 76
... Answer is C
Explanation: Average after 11 innings = 24 Required number of runs = (24 * 11) – (20 * 10) = 264 – 200 = 64
A. 12
B. 42
C. 64
D. 76
... Answer is C
Explanation: Average after 11 innings = 24 Required number of runs = (24 * 11) – (20 * 10) = 264 – 200 = 64
24. Find the average of all the numbers between 11 and 36 which are divisible by 5.
,
A. 15
B. 20
C. 25
D. 30
... Answer is C
Explanation: Average = (15 + 20 + 25 + 30 + 35) / 5 = 125/5 = 25
A. 15
B. 20
C. 25
D. 30
... Answer is C
Explanation: Average = (15 + 20 + 25 + 30 + 35) / 5 = 125/5 = 25
25. The average of five consecutive odd numbers is 51. What is the difference between the highest and lowest number?
,
A. 3
B. 7
C. 8
D. 11
... Answer is C
Explanation: Let the numbers be x, x + 2, x + 4, x + 6 and x + 8. Then, [x + (x + 2) + (x + 4) + (x + 6) + (x + 8)] / 5 = 51 5x + 20 = 255 x = 47 So, required difference = (47 + 8) – 47 = 8
A. 3
B. 7
C. 8
D. 11
... Answer is C
Explanation: Let the numbers be x, x + 2, x + 4, x + 6 and x + 8. Then, [x + (x + 2) + (x + 4) + (x + 6) + (x + 8)] / 5 = 51 5x + 20 = 255 x = 47 So, required difference = (47 + 8) – 47 = 8
26. Find the average of first 30 natural numbers.
,
A. 12
B. 15
C. 15.5
D. 16
... Answer is C
Explanation: Sum of first n natural numbers = n(n+1)/2 Hence, sum of first 30 natural numbers = (30 x 31)/2 = 465 Therefore, required average of = 465/30 = 15.5
A. 12
B. 15
C. 15.5
D. 16
... Answer is C
Explanation: Sum of first n natural numbers = n(n+1)/2 Hence, sum of first 30 natural numbers = (30 x 31)/2 = 465 Therefore, required average of = 465/30 = 15.5
27. The average weight of three boys P, Q and R is 54 kg, while the average weight of three boys Q, S and T is 60 kg. What is the average weight of P, Q, R, S and T?.
,
A. 66.4 kg
B. 63.2 kg
C. 58.8kg
D. None of these
... Answer is D
Explanation: Total weight of (P + Q + R) = {54*3} kg = 162 kg Total weight of(Q + S + T) = (60 *3) kg = 180 kg Adding both, we get : P + 2Q + S + R + T = (162 + 180) kg = 342 kg So, to find the average weight of P, Q, R, S & T, we ought to know Q's weight, which is not given. The data is inadequate.
A. 66.4 kg
B. 63.2 kg
C. 58.8kg
D. None of these
... Answer is D
Explanation: Total weight of (P + Q + R) = {54*3} kg = 162 kg Total weight of(Q + S + T) = (60 *3) kg = 180 kg Adding both, we get : P + 2Q + S + R + T = (162 + 180) kg = 342 kg So, to find the average weight of P, Q, R, S & T, we ought to know Q's weight, which is not given. The data is inadequate.
28. If the sum of three consecutive even numbers is 44 more than the average of these numbers, then the largest of these numbers is?
,
A. 20
B. 24
C. 22
D. None of these
... Answer is B
Explanation: Let the smallest of these number be x. The other two numbers are (x + 2) and (x + 4). x + (x + 2) + (x + 4) = (X + (X+2) + (x+4)) / 3 + 44 3x + 3*(x + 2) + 3*(x + 4) = x + (x + 2) + (x + 4) + 132 9x + 18 = 3x + 138 6x = 120 x = 20 Therefore, the largest number is 24.
A. 20
B. 24
C. 22
D. None of these
... Answer is B
Explanation: Let the smallest of these number be x. The other two numbers are (x + 2) and (x + 4). x + (x + 2) + (x + 4) = (X + (X+2) + (x+4)) / 3 + 44 3x + 3*(x + 2) + 3*(x + 4) = x + (x + 2) + (x + 4) + 132 9x + 18 = 3x + 138 6x = 120 x = 20 Therefore, the largest number is 24.
29. If from a group of 5 people, an old member is replaced by a new one, the average age is same as it was 3 years ago. What is the difference between the ages of the old member and the new one?
,
A. 12
B. 15
C. 11
D. 18
... Answer is B
Explanation: The present average age is (x + 3) when the old member is present and it equals to x when an old member is replaced by a new one. The difference in the ages of the old member and the new one is equal to the difference in the total age before and after the replacement = 15 years.
A. 12
B. 15
C. 11
D. 18
... Answer is B
Explanation: The present average age is (x + 3) when the old member is present and it equals to x when an old member is replaced by a new one. The difference in the ages of the old member and the new one is equal to the difference in the total age before and after the replacement = 15 years.
30. The batting average of a particular batsman is 60 runs in 46 innings. If the difference in his highest and lowest score is 150 runs and his average excluding these two innings is 58 runs, find his highest score
,
A. 179
B. 208
C. 210
D. None of the above
... Answer is A
Explanation: Total runs scored by the batsman = 60*46 = 2760 runs Now excluding the two innings the runs scored = 58*44 = 2552 runs Hence the runs scored in the two innings = 2760 – 2552 = 208 runs. Let the highest score be x, hence the lowest score = x – 150 x + (x - 150) = 208 2x = 358 x = 179 runs
A. 179
B. 208
C. 210
D. None of the above
... Answer is A
Explanation: Total runs scored by the batsman = 60*46 = 2760 runs Now excluding the two innings the runs scored = 58*44 = 2552 runs Hence the runs scored in the two innings = 2760 – 2552 = 208 runs. Let the highest score be x, hence the lowest score = x – 150 x + (x - 150) = 208 2x = 358 x = 179 runs
31. Vijay says that his weight is between 75kg and 85 kg. But his younger sister disagrees with Vijay and says that his weight is greater than 70kg but less than 80 kg. Vijay’s mother says that his weight cannot be greater than 78 kg. If all the three predictions are correct, what is the average of different probable weights of Vijay?
,
A. 75
B. 80
C. 78.5
D. 76.5
... Answer is D
Explanation: Vijay’s opinion: - 75 < Vijay’s age < 85 Sister’s opinion: - 72 < Vijay’s age < 82 Mother’s opinion: - Vijay’s age < 78 As all the above three conditions are true, the values satisfying them are 76 & 77. Average value = (76 + 77)/ 2 = 76.5
A. 75
B. 80
C. 78.5
D. 76.5
... Answer is D
Explanation: Vijay’s opinion: - 75 < Vijay’s age < 85 Sister’s opinion: - 72 < Vijay’s age < 82 Mother’s opinion: - Vijay’s age < 78 As all the above three conditions are true, the values satisfying them are 76 & 77. Average value = (76 + 77)/ 2 = 76.5
32. Average score for Virat Kohli in a series of 10 matches is 38.9 runs. If the average for first six matches comes out to be 42 what is his average in the last 4 matches of the series?
,
A. 34.25
B. 35
C. 33.25
D. 34.25
... Answer is D
Explanation: Average runs scored by Virat Kohli in 10 matches: Total runs scored/10—(1) Average runs scored by Virat Kohli in 6 matches: (Total runs in 6 matches)/6 => 42 = Runs/6 =>252 runs Using (1): =>38.9 = Runs/10 =>389 runs Runs scored in rest 4 matches: 389 - 252 runs => 137 runs Average runs scored by Virat Kohli in 4 matches: 137/4 = 34.25 runs
A. 34.25
B. 35
C. 33.25
D. 34.25
... Answer is D
Explanation: Average runs scored by Virat Kohli in 10 matches: Total runs scored/10—(1) Average runs scored by Virat Kohli in 6 matches: (Total runs in 6 matches)/6 => 42 = Runs/6 =>252 runs Using (1): =>38.9 = Runs/10 =>389 runs Runs scored in rest 4 matches: 389 - 252 runs => 137 runs Average runs scored by Virat Kohli in 4 matches: 137/4 = 34.25 runs
33. The average of 5 quantities is 6. The average of 3 of them is 4. What is the average of remaining 2 numbers?
,
A. 9
B. 10
C. 8
D. 9.5
... Answer is A
Explanation: Not Available
A. 9
B. 10
C. 8
D. 9.5
... Answer is A
Explanation: Not Available
34. The average weight of 8 persons is increased by 2.5 kg when one of them who weighs 56 kg is replaced by a new man. The weight of the new man is
,
A. 76 years
B. 72 years
C. 78 years
D. None of these
... Answer is A
Explanation: Not Available
A. 76 years
B. 72 years
C. 78 years
D. None of these
... Answer is A
Explanation: Not Available
35. Average of six numbers comes out to be 3.95. Average of two of the numbers is 3.4 while the average of other two is 3.85. Determine the average of the two numbers left.
,
A. 4.7
B. 4.8
C. 4.3
D. 4.6
... Answer is D
Explanation: In the given question we have taken average of 6 numbers taken 2 at a time which makes 3 numbers. => 3.95 (Average of 1st and 2nd number) => 3.85 (Average of 3rd and 4th number) => 3.4 (Average of 5th and 6th number) 3.95 = (3.4 + 3.85 + x)/3 x = 4.6
A. 4.7
B. 4.8
C. 4.3
D. 4.6
... Answer is D
Explanation: In the given question we have taken average of 6 numbers taken 2 at a time which makes 3 numbers. => 3.95 (Average of 1st and 2nd number) => 3.85 (Average of 3rd and 4th number) => 3.4 (Average of 5th and 6th number) 3.95 = (3.4 + 3.85 + x)/3 x = 4.6
36. The average of 5 quantities is 6. The average of 3 of them is 4. What is the average of remaining 2 numbers?
,
A. 9
B. 10
C. 8
D. 9.5
... Answer is A
Explanation: No explanation is available for this question!
A. 9
B. 10
C. 8
D. 9.5
... Answer is A
Explanation: No explanation is available for this question!
37. Determine a man’s present age if his present age is 125% of his age 10 years ago and 83.333% of his age 10 years from now.
,
A. 40 years
B. 50 years
C. 60 years
D. 70 years
... Answer is B
According to the question current age of the man is: 1. 125% of his age 10 years ago 2. 83.333% of his age 10 years from now Let the current age of the man be x. Therefore, equating the two equations generated: 125/100(x-10) = 250/3(x+10) x = 50
A. 40 years
B. 50 years
C. 60 years
D. 70 years
... Answer is B
According to the question current age of the man is: 1. 125% of his age 10 years ago 2. 83.333% of his age 10 years from now Let the current age of the man be x. Therefore, equating the two equations generated: 125/100(x-10) = 250/3(x+10) x = 50
38. A batsman makes a score of 87 runs in the 17th match and thus increases his average by 3. Find his average after 17th match
,
A. 36
B. 37
C. 38
D. 39
... Answer is D
Explanation: Let the average after 17th match is x then the average before 17th match is x-3 so 16(x-3) + 87 = 17x => x = 87 - 48 = 39
A. 36
B. 37
C. 38
D. 39
... Answer is D
Explanation: Let the average after 17th match is x then the average before 17th match is x-3 so 16(x-3) + 87 = 17x => x = 87 - 48 = 39
39. Average weight of 10 people increased by 1.5 kg when one person of 45 kg is replaced by a new man. Then weight of the new man is
,
A. 50
B. 55
C. 60
D. 65
... Answer is C
Explanation: Total weight increased is 1.5 * 10 = 15. So weight of new person is 45+15 = 60
A. 50
B. 55
C. 60
D. 65
... Answer is C
Explanation: Total weight increased is 1.5 * 10 = 15. So weight of new person is 45+15 = 60
40. Average of five numbers is 27. If one number is excluded the average becomes 25. The excluded number is
,
A. 35
B. 45
C. 55
D. 65
... Answer is A
Explanation: Number is (5*27) - (4*25) = 135-100 = 35
A. 35
B. 45
C. 55
D. 65
... Answer is A
Explanation: Number is (5*27) - (4*25) = 135-100 = 35
41. Average of 10 matches is 32, How many runs one should should score to increase his average by 4 runs.
,
A. 76
B. 76
C. 78
D. 80
... Answer is B
Explanation: Average after 11 innings should be 36 So, Required score = (11 * 36) - (10 * 32) = 396 - 320 = 76
A. 76
B. 76
C. 78
D. 80
... Answer is B
Explanation: Average after 11 innings should be 36 So, Required score = (11 * 36) - (10 * 32) = 396 - 320 = 76
42. The average age of husband, wife and their child 3 years ago was 27 years and that of wife and the child 5 years ago was 20 years. The present age of the husband is
,
A. 40
B. 35
C. 45
D. 55
... Answer is A
Explanation: Sum of the present ages of husband, wife and child = (27 * 3 + 3 * 3) years = 90 years. Sum of the present ages of wife and child = (20 * 2 + 5 * 2) years = 50 years. Husband's present age = (90 - 50) years = 40 years
A. 40
B. 35
C. 45
D. 55
... Answer is A
Explanation: Sum of the present ages of husband, wife and child = (27 * 3 + 3 * 3) years = 90 years. Sum of the present ages of wife and child = (20 * 2 + 5 * 2) years = 50 years. Husband's present age = (90 - 50) years = 40 years
43. The average age of the mother and her six children is 12 years which is reduced by 5 years if the age of the mother is excluded. How old is the mother
,
A. 40
B. 41
C. 42
D. 43
... Answer is C
Explanation: Not Available
A. 40
B. 41
C. 42
D. 43
... Answer is C
Explanation: Not Available
44. The average weight of a class of 24 students is 35 kg. If the weight of the teacher be included, the average rises by 400 g. The weight of the teacher is
,
A. 45
B. 50
C. 55
D. 60
... Answer is A
Explanation: Not Available
A. 45
B. 50
C. 55
D. 60
... Answer is A
Explanation: Not Available
45. A grocer has a sale of Rs. 6435, Rs. 6927, Rs. 6855, Rs. 7230 and Rs. 6562 for 5 consecutive months. How much sale must he have in the sixth month so that he gets an average sale of Rs. 6500?
,
A. Rs. 4991
B. Rs. 5991
C. Rs. 6001
D. Rs. 6991
... Answer is A
Explanation: Total sale for 5 months = Rs. (6435 + 6927 + 6855 + 7230 + 6562) = Rs. 34009. Required sale = Rs. [ (6500 x 6) - 34009 ] = Rs. (39000 - 34009) = Rs. 4991.
A. Rs. 4991
B. Rs. 5991
C. Rs. 6001
D. Rs. 6991
... Answer is A
Explanation: Total sale for 5 months = Rs. (6435 + 6927 + 6855 + 7230 + 6562) = Rs. 34009. Required sale = Rs. [ (6500 x 6) - 34009 ] = Rs. (39000 - 34009) = Rs. 4991.
46. The average monthly income of P and Q is Rs. 5050. The average monthly income of Q and R is Rs. 6250 and the average monthly income of P and R is Rs. 5200. The monthly income of P is
,
A. 3500
B. 4000
C. 4050
D. 5000
... Answer is B
Explanation: Let P, Q and R represent their respective monthly incomes. Then, we have: P + Q = (5050 x 2) = 10100 .... (i) Q + R = (6250 x 2) = 12500 .... (ii) P + R = (5200 x 2) = 10400 .... (iii) Adding (i), (ii) and (iii), we get: 2(P + Q + R) = 33000 or P + Q + R = 16500 .... (iv) Subtracting (ii) from (iv), we get P = 4000. P's monthly income = Rs. 4000.
A. 3500
B. 4000
C. 4050
D. 5000
... Answer is B
Explanation: Let P, Q and R represent their respective monthly incomes. Then, we have: P + Q = (5050 x 2) = 10100 .... (i) Q + R = (6250 x 2) = 12500 .... (ii) P + R = (5200 x 2) = 10400 .... (iii) Adding (i), (ii) and (iii), we get: 2(P + Q + R) = 33000 or P + Q + R = 16500 .... (iv) Subtracting (ii) from (iv), we get P = 4000. P's monthly income = Rs. 4000.
47. Find the average of numbers 87, 84, 86, 90, 82, 88, 78.
,
A. 85
B. 84
C. 83
D. 82
... Answer is A
The sum of all the observations here is 87 + 84 + 86 + 90 +82 + 88 +78 = 595 Number of observations = 7 So, Average = 595/7 = 85
A. 85
B. 84
C. 83
D. 82
... Answer is A
The sum of all the observations here is 87 + 84 + 86 + 90 +82 + 88 +78 = 595 Number of observations = 7 So, Average = 595/7 = 85
48. The average of 4 terms is 20 and the 1st term is 1/3 of the remaining terms. What will be the first number?
,
A. 30
B. 20
C. 60
D. 80
... Answer is B
Average of 4 terms = 20 Hence, the total sum of 4 terms = 80 Let terms be A,B,C,D So, the sum will be A+B+C+D =80 Given, 3A = B+C+D So, 4A = 80, A = 20
A. 30
B. 20
C. 60
D. 80
... Answer is B
Average of 4 terms = 20 Hence, the total sum of 4 terms = 80 Let terms be A,B,C,D So, the sum will be A+B+C+D =80 Given, 3A = B+C+D So, 4A = 80, A = 20
49. In a hotel, the tariff for every odd dates is Rs.1000 and for even dates is Rs. 2000. If the man paid total of 30000 in all. For how many days did he stay in the hotel given that the first day is 5th date of the month?
,
A. 50
B. 20
C. 40
D. 60
... Answer is B
Total tariff = 30000 So, for odd dates (5th , 7th , and so on) = 1000 And for even dates (6th , 8th and so on ) = 2000 So, the average amount of money for 2 days is Rs. 1500. So, total amount paid = 30000 So , number of days he stayed in the hotel = 30000/1500 = 20.
A. 50
B. 20
C. 40
D. 60
... Answer is B
Total tariff = 30000 So, for odd dates (5th , 7th , and so on) = 1000 And for even dates (6th , 8th and so on ) = 2000 So, the average amount of money for 2 days is Rs. 1500. So, total amount paid = 30000 So , number of days he stayed in the hotel = 30000/1500 = 20.
50. For 9 innings, Roman has an average of 65 runs. In the tenth inning, he scores 200 runs, thus increasing his average . His average increased by
,
A. 78.5
B. 72
C. 13.5
D. 77.5
... Answer is C
Total score for 65 innings = 65×9 = 585 Total score after 10th innings = 585 + 200 = 785 So, the new average is 785/10 = 78.5 So, the increment is of 13.5
A. 78.5
B. 72
C. 13.5
D. 77.5
... Answer is C
Total score for 65 innings = 65×9 = 585 Total score after 10th innings = 585 + 200 = 785 So, the new average is 785/10 = 78.5 So, the increment is of 13.5






![FR & SR and Financial Hand Book for IPO Examination [Online Test]-IPO2021](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEgcp_wSYLoI1j137GQIf6WXaW7CaVIWml96tfINUIcenirpM1HqVJXJUUoCQwjTxiubiTYMYavQXjcH5mZAK5bqHGK2UY9tODpu0w2ItUGrVSbGEjFht-KGRxRAxivDZhtuMojm8qZ6gUTV/s72-w400-c-h170/online-test-series.jpg)

![IT Modernization Project 2012 and Updates MCQ:- MCQ on IT Modernization Project 2012 and Updates [IPO 2021]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEi7eD-21TRwFFlBF0eRKjj99G9D1SJW2Vc7mHwbzoMVJDwjekV6IXlcZxwzbU_ZG-4Q5WgHjRNUrkl_jalsinJOxAAlZjchdXGi9RO8i1EDkkSIYJBgr3KUN9zj0nJUdIuQ0jrQaMWslYWh/s72-c/online-test-series.jpg)


No comments
Leave a Reply: Your email address will not be published.